Question

An NBA fan named Mark claims that there are more fouls called on his team 1 point

any other team, but the commissioner says that the number of fouls called
against his team are no different than any other team. Mark finds that the
average number of fouls in any game in the NBA is 11.5. He takes a random
sample of 34 of games involving his team and finds that there are an
average of 12.2 fouls against his team, with a standard deviation of 1.6 fouls.
What is the correct conclusion? Use a = 0.05

a) The p value is 2.55 indicating insufficient evidence for his claim.

b)The p-value is 0.008, indicating sufficient evidence for his claim.

c)The p-value is 0.008, indicating insufficient evidence for his claim.

d)The p-value is 2.55, indicating sufficient evidence for his claim.

Answer 1

`t=(12.2-11.5)/((1.6)/(√(34)))=2.551`

`df = n-1=34-1=33`

`p_v =P(t_((33))>2.551)=0.008`

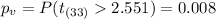
Since the p value is less than the significance level of 0.05 we have enough evidence to reject the null hypothesis in favor of the claim

And the best conclusion for this case would be:

b)The p-value is 0.008, indicating sufficient evidence for his claim.

Explanation:

Information provided

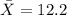`\bar X=12.2` represent the sample mean fould against

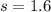`s=1.6` represent the sample standard deviation

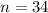`n=34` sample size

represent the value that we want to test

`\alpha=0.05` represent the significance level for the hypothesis test.

t would represent the statistic (variable of interest)

`p_v` represent the p value for the test (variable of interest)

System of hypothesis

We need to conduct a hypothesis in order to check if the true mean is higher than 11.5 fouls per game:

Null hypothesis:
`\mu \leq 11.5`

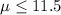
Alternative hypothesis:
`\mu > 11.5`

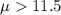
The statistic is given by:

`t=(\bar X-\mu_o)/((s)/(√(n)))` (1)

The statistic is given by:

`t=(12.2-11.5)/((1.6)/(√(34)))=2.551`

P value

The degreed of freedom are given by:

`df = n-1=34-1=33`

Since is a one side test the p value would be:

`p_v =P(t_((33))>2.551)=0.008`

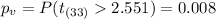
Since the p value is less than the significance level of 0.05 we have enough evidence to reject the null hypothesis in favor of the claim

And the best conclusion for this case would be:

b)The p-value is 0.008, indicating sufficient evidence for his claim.