Question

The population of a certain species of fish has a growth rate of 2.2% per year. It is estimated that the the current population is 350,000. Estimate the number of years it will take the fish population to reach 1,000,000. Round your answer to the nearest tenth.

Answer 1

47.7

Explanation:

right answer

Answer 2

We have been given that the population of a certain species of fish has a growth rate of 2.2% per year. It is estimated that the the current population is 350,000.

We are asked to find the time it will take the fish population to reach 1,000,000.

We will use exponential growth formula to solve our given problem.

An exponential growth function is in form
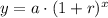`y=a\cdot (1+r)^x`, where,

y = Final amount,

a = Initial amount,

r = Growth rate in decimal form,

x = Time

`2.2\%=(2.2)/(100)=0.022`

`y=350,000\cdot (1+0.022)^x`

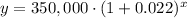
To find the time for the fish population to reach 1,000,000, we will substitute
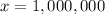`x=1,000,000` in our equation as:

`1,000,000=350,000\cdot (1+0.022)^x`

`1,000,000=350,000\cdot (1.022)^x`

`(1,000,000)/(350,000)=(350,000\cdot (1.022)^x)/(350,000)`

`2.8571428571428571=(1.022)^x`

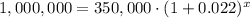
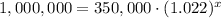
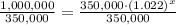
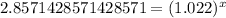
Now we will take natural log on both sides:

`\text{ln}(2.8571428571428571)=\text{ln}((1.022)^x)`

`\text{ln}(2.8571428571428571)=x\cdot \text{ln}(1.022)`

`x=\frac{\text{ln}(2.8571428571428571)}{\text{ln}(1.022)}`

`x=(1.0498221244986776733)/(0.0217614917815127)`

`x=48.2421947`

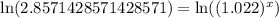
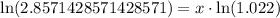
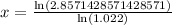
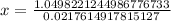
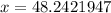
Upon rounding to nearest tenth, we will get:

`x\approx 48.2`

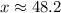
Therefore, it will take approximately 48.2 years for the fish population to reach 1,000,000.