Question

A local brewery produces three premium lagers named Half Pint, XXX, and Dark Night. Of its premium lagers, they bottle 40% Half Pint, 40% XXX, and 20% Dark Night lagers. In a marketing test of a sample of consumers, 36 preferred the Half Pint lager, 35 preferred the XXX lager, and 9 preferred the Dark Night lager. Using a chi-square goodness-of-fit test, decide to retain or reject the null hypothesis that production of the premium lagers matches these consumer preferences using a 0.05 level of significance.

a. State the value of the test statistic.

b. Retain or reject the null hypothesis?

Answer 1

(a) The test statistic value is, 5.382.

(b) Retain the null hypothesis.

Explanation:

A Chi-square test for goodness of fit will be used in this case.

The hypothesis can be defined as:

H₀: The observed frequencies are same as the expected frequencies.

Hₐ: The observed frequencies are not same as the expected frequencies.

The test statistic is given as follows:

`\chi^(2)=\sum\limits^(n)_(i=1){((O_(i)-E_(i))^(2))/(E_(i))}`

The information provided is:

Observed values:

Half Pint: 36

XXX: 35

Dark Night: 9

TOTAL: 80

The expected proportions are:

Half Pint: 40%

XXX: 40%

Dark Night: 20%

Compute the expected values as follows:

E (Half Pint)
`=(40)/(100)* 80=32`

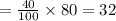
E (XXX)
`=(40)/(100)* 80=32`

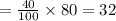
E (Dark night)
`=(20)/(100)* 80=16`

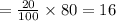
Compute the test statistic as follows:

`\chi^(2)=\sum\limits^(n)_(i=1){((O_(i)-E_(i))^(2))/(E_(i))}`

`=[((36-32)^(2))/(32)]+[((35-32)^(2))/(32)]+[((9-16)^(2))/(16)]`

`=3.844`

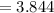
The test statistic value is, 5.382.

The degrees of freedom of the test is:

n - 1 = 3 - 1 = 2

The significance level is, α = 0.05.

Compute the p-value of the test as follows:

p-value = 0.1463

*Use a Ch-square table.

p-value = 0.1463 > α = 0.05.

So, the null hypothesis will not be rejected at 5% significance level.

Thus, concluding that the production of the premium lagers matches these consumer preferences.