Question

A toy boat is bobbing in the water.

Its distance D(t) (in m) from the floor of the lake as a function of time t (in seconds) can be modeled by a sinusoidal expression of the form a×sin(b×t) + d.

At t=0, when the boat is exactly in the middle of its oscillation, it is 1 m above the water's floor. The boat reaches its maximum height of 1.2 m after pi/4 seconds.

Find D(t).

Answer 1

0.2sin(2t)+1

Explanation:

This is a simplified version of the answer above me.

Answer 2

`D(t) = 1\,m + (0.2\,m)\cdot \sin \left[\right(2\,(rad)/(s) \left)\cdot t\right]`

Explanation:

The sinusoidal expression has the following form:

`D(t) = D+A\cdot \sin (B\cdot t)`

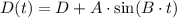
Where:

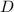`D` - Initial distance from the floor of the lake, in meters.

`A` - Amplitude of oscillation, in meters.

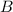`B` - Angular frequency, in radians.

Now, each coefficient is derived as follows:

Initial distance from the floor of the lake

`D = 1\,m`

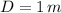
Amplitude of oscillation

`A = 1.2\,m - 1\,m`

`A = 0.2\,m`

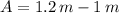
Angular frequency

From the statement it is known that boat reaches its maximum height in a quarter of its oscillation. Then, the angular frequency is:

`B = ((1)/(2)\pi \,rad)/((1)/(4)\pi \,s)`

`B = 2\,(rad)/(s)`

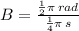
The expression is:

`D(t) = 1\,m + (0.2\,m)\cdot \sin \left[\right(2\,(rad)/(s) \left)\cdot t\right]`