Answer:
A and B
Explanation:
We are given that


Compare with it
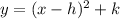
Where vertex=(h,k)
We get
Vertex of g=(2,-1)
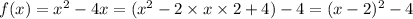
Vertex of f=(2,-4)
Equation of axis of symmetry=x-coordinate of vertex
Axis of symmetry of g
x=2
Axis of symmetry of f
x=2
Differentiate w.r.t x
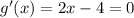
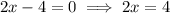

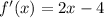
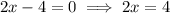

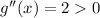
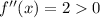
f and g have both minima at x=2
Hence, option A and B are true.