Answer:
(a)
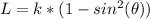
(b) L reaches its maximum value when θ = 0 because cos²(0) = 1
Explanation:
Lambert's Law is given by:
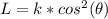 (1)
(1)
(a) We can rewrite the above equation in terms of sine function using the following trigonometric identity:
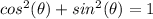
 (2)
(2)
By entering equation (2) into equation (1) we have the equation in terms of the sine function:
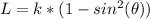
(b) When θ = 0, we have:
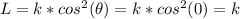
We know that cos(θ) is a trigonometric function, between 1 and -1 and reaches its maximun values at nπ, when n = 0,1,2,3...
Hence, L reaches its maximum value when θ = 0 because cos²(0) = 1.
I hope it helps you!