Question

Tamara finds the sum of two number cubes rolled at the same time. The chart below shows all possible sums from the 36 possible combinations when rolling two number cubes. How many times should Tamara expect the sum of the two cubes to be equal to 5 if she rolls the two number cubes 180 times?

Answer 1

Answer: 10 times.

Step-by-step explanation: According to the chart, there is only one combination that results in a sum of 5, which is (1,4) or (4,1). So the probability of getting a sum of 5 in one roll is 2/36 or 1/18.

If Tamara rolls the two number cubes 180 times, she can expect to get a sum of 5 approximately (1/18) x 180 = 10 times.

Answer 2

Tamara should expect the sum of the two cubes to be equal to 5 20 times.

Explanation:

The sample space of rolling a number cube are:

S = {1, 2, 3, 4, 5, 6}

If two such cubes are rolled together, then the sum of the two cubes will be 5 for the combinations below:

S₁ = {(1, 4), (2, 3), (3, 2) and (4, 1)}

The total number of outcomes will be, N = 36.

Compute the probability that the sum of rolling two numbered cubes as follows:

`P(\text{Sum}=5)=(4)/(36)=(1)/(9)`

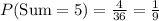
Let X = number of time the sum of the two numbers on two cubes is 5.

Two numbered cubes are rolled n = 180 times.

The event of getting a sum of 5 in independent of the other results.

The random variable X follows a Binomial distribution with parameters n = 180 and p =
`(1)/(9)`.

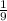The expected value of X is:

`E(X)=np`

Compute the expected number of times Tamara expects the sum of the two cubes to be equal to 5 as follow:

`E(X)=np\\=180* (1)/(9)\\=20`

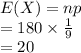
Thus, Tamara should expect the sum of the two cubes to be equal to 5 20 times.