Answer:
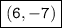
Explanation:
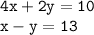
First Let's solve for x in x-y=13
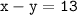
Add y to both sides:
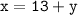
Now, Substitute x=13+y into 4x+2y=10:-
Let x=13+y
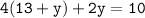

Now, let's solve for y in 52+6y=10:-

Subtract 52 from both sides:-
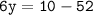
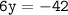
Divide both sides by 6:-
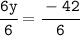

Now, we'll Substitute y=-7 into to x=13+y to find "x":-
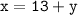

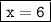
___