Answer:
Both value of V are different, therefore, what is proposed in the question is not possible
Step-by-step explanation:
Given data:
E₁=35 GPa (longitudinal moduli of elasticity)
E₂=5.17 GPa (transverse moduli of elasticity)
Ee=3.4 GPa (elastic modulus epoxi)
Ef=131 GPa (elastic modulus fiber)
To produce a continuous and oriented aramid fiber-epoxy matrix, the volume fraction of the fibers must be the same. Then, we must calculate the volume fraction of the fibers in the longitudinal and transverse:
For the longitudinal:
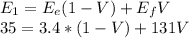
Solving for V:
V=0.2476
For the transverse:
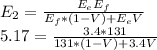
Solving for V:
V=0.3515
You can see that both value of V are different, therefore, what is proposed in the question is not possible