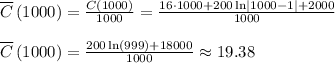Question

The weekly marginal cost of producing x pairs of tennis shoes is given by the following function, where C(x) is cost in dollars.

C'(x)= 16+ (200/(x-1))

If the fixed costs are $2,000 per week, find the cost function. C(x)=??

What is the average cost per pair of shoes if 1,000 pairs of shoes are produced each week?

Answer 1

The cost function is
`C(x)=16x+200\ln \left|x-1\right|+2000`.

The average cost of 1000 shoes is $19.38.

Explanation:

We define the marginal cost function to be the derivative of the cost function or
`C'\left( x \right)`.

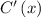To find the cost function,
`C(x)` we need to integrate the marginal cost function

`\int {C'(x)} \, dx =C(x)\\\\\int \:16+(200)/(x-1)dx\\\\\mathrm{Apply\:the\:Sum\:Rule}:\quad \int f\left(x\right)\pm g\left(x\right)dx=\int f\left(x\right)dx\pm \int g\left(x\right)dx\\\\=\int \:16dx+\int (200)/(x-1)dx`

`\int \:16dx=16x`

`\int (200)/(x-1)dx=200\ln \left|x-1\right|`

`\mathrm{Add\:a\:constant\:to\:the\:solution}\\\\C(x)=\int \:16+(200)/(x-1)dx=16x+200\ln \left|x-1\right|+D`

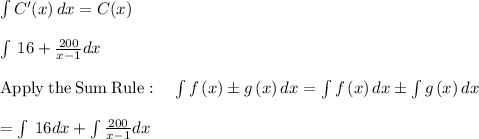
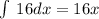
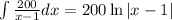
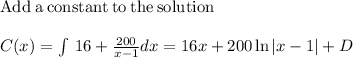
We know that the fixed costs are $2,000 per week, so the constant
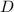`D` is equal to 2000, and the cost function is

`C(x)=16x+200\ln \left|x-1\right|+2000`

If
`C(x)` is the cost function for some item then the average cost function is,

`\overline{C}\left( x \right) = \frac{{C\left( x \right)}}{x}`

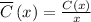
We know that 1,000 pairs of shoes are produced each week, so the the average cost is

`\overline{C}\left( 1000 \right) = \frac{{C\left( 1000 \right)}}{1000}=(16\cdot 1000+200\ln \left|1000-1\right|+2000)/(1000) \\\\\overline{C}\left( 1000 \right)=(200\ln \left(999\right)+18000)/(1000)\approx19.38`