Question

In triangle ABC, angles A and angle B are complementary, where cos A = 0.5.

What is the measure, in degrees, of angle B?

Hint: first find cos^-1 (.5), then find the complement of this angle.

60 degrees

30 degrees

90 degrees

0 degrees

Answer 1

∴∠B=30°

Explanation:

Complementary:

Two angles is said to complementary when the sum of two angles is 90 degree.

Given that,

∠A and ∠B are complementary.

Then, ∠A + ∠B = 90°

Cos A = 0.5

`\Rightarrow A=cos^(-1)(0.5)`

`\Rightarrow A=cos^(-1)(cos \ 60^\circ)`

`\Rightarrow A=60^\circ`

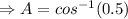
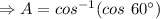
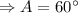
Since

∠A + ∠B = 90°

⇒ 60°+ ∠B = 90° [ plug ∠A= 60°]

⇒∠B=90°-60°

⇒∠B=30°

∴∠B=30°