Question

The brick wall (of thermal conductivity 0.81 W/m · ◦ C) of a building has dimensions of 6.2 m by 12 m and is 8 cm thick. How much heat flows through the wall in a 16.9 h period when the average inside and outside temperatures are, respectively, 30◦C and 8◦C? Answer in units of MJ.

Answer 1

the amount of energy flowing is 1.008x10⁹J

Step-by-step explanation:

To calculate how much heat flows, the expression is the following:

`E=(KA*delta-T)/(L) *t`

Where

K=thermal conductivity=0.81W/m°C

A=area=6.2*12=74.4m²

ΔT=30-8=22°C

L=thickness=8cm=0.08m

t=time=16.9h=60840s

Replacing:

`E=(0.81*74.4*22)/(0.08) *60840=1.008x10^(9) J`

Answer 2

The heat flows through the wall is 1008.3 MJ

Step-by-step explanation:

Given;

thermal conductivity, K = 0.81 W/m · ◦ C

dimension of the brick wall, = 6.2 m by 12 m

thickness of the brick wall, L = 8 cm = 0.08 m

period of heat flow, t = 16.9 h

temperature change, Δθ = 30◦C - 8◦C = 22°C

Power generated by the brick wall:

`P = (KA \delta \theta)/(L)`

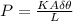
where;

A is the area of the brick wall

A = 6.2 m x 12 m = 74.4 m²

`P = (KA \delta \theta)/(L) =(0.81*74.4*22)/(0.08) =16,572.6 \ W`

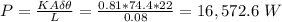
Heat flow through the wall:

E = Pt

where;

t is period of flow

t = 16.9 h = 16.9h x 60 mins x 60 sec = 60840 seconds

E = 16572.6 x 60840 = 1008276984 J

E = 1008.3 x 10⁶ J

E = 1008.3 MJ

Therefore, the heat flows through the wall is 1008.3 MJ