Question

there is a rectangle abcd.the length of the rectangle is increased by 10%.the width of the rectangle is increased by 5%,what is the percentage increase in the recatangle.

Answer 1

Explanation:

Answer 2

Therefore the area of rectangle is increased by 15.5%.

Explanation:

Assume the length and width of the rectangle be x and y respectively.

The area of the rectangle is = length×width

=xy.

Now the length of the rectangle is increased by 10%.

Then the length of the rectangle increased =
`x* (10)/(100)`.

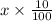New length of the rectangle is
`=x+(10x)/(100)`

`=(100x+10x)/(100)`

`=(110x)/(100)`

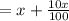
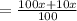
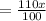
The width of the rectangle is increased by 5%.

Then the width of the rectangle increased =
`y* (5)/(100)`.

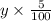New length of the rectangle is
`=y+(5y)/(100)`

`=(100y+5y)/(100)`

`=(105y)/(100)`

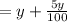
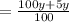
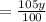
New area of the rectangle is =
`(110x)/(100)*(105y)/(100)`

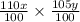
= 1.155 xy.

The percentage of area increase is

`=\frac{\textrm{New area - Original area}}{\textrm{Original area}}* 100`

`=(1.155xy-xy)/(xy)* 100`

`=(0.155xy)/(xy)* 100`

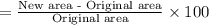
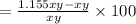
=15.5.

Therefore the area of rectangle is increased by 15.5%.