Question

A 70 kg human body typically contains 140 g of potassium. Potassium has a chemical atomic mass of 39.1 u and has three naturally occurring isotopes. One of those isotopes, 40K, is radioactive with a half-life of 1.3 billion years and a natural abundance of 0.012%. Each 40K decay deposits, on average, 1.0 MeV of energy into the body.

Part A What yearly dose in Gy does the typical person receive from the decay of 40K in the body? Express your answer using two significant figures.

Q=________Gy

Answer 1

The typical person receives
`3.15 * 10^(-4) \text{ Gray}\)` from the decay of 40K in the body.

How to determine this?

Given:

`\(N =\)` number of atoms of natural
`\(K = \left(\frac{140 \text{ g} * 6.0221367 * 10^(23) \text{ atoms/mole}}{39.1 \text{ g/mole}}\right) = 2.1562638 * 10^(24)\)` atoms

`\(N_o =\) number of atoms of \(K-40\) initially \(= (0.00012) * N = 2.5875165 * 10^(20)\) atoms`

The formula for the number of atoms of \(K-40\) remaining after time \(t\) is:

`\[N_f = N_o * e^(-\lambda t)\]`

where
`\(\lambda = (\ln 2)/(t_(1/2)) = \frac{\ln 2}{1.3 * 10^9 \text{ yr}} = 5.3319014 * 10^(-10) \text{ yr}^(-1)\)`

Given
`\(t = 1 \text{ yr}\)`, this yields
`\(N_f = 2.587516499 * 10^(20)\)` atoms.

The difference between
`\(N_o\)` and
`\(N_f\)` is approximately
`\(1.38 * 10^(11)\)` atoms, representing the number of
`\(K-40\)` atoms decaying in a period of 1 year.

Each decaying
`\(K-40\)` atom deposits
`\(1 \text{ MeV}\)` of energy. Thus, the total energy deposited in the human body during the year is
`\(1.38 * 10^(11) \text{ MeV}\) or \(1.38 * 10^(17) \text{ eV}\)`.

Converting this energy to Joules:
`\((1.38 * 10^(17) \text{ eV}) * (1.6 * 10^(-19) \text{ J/eV}) = 0.02208 \text{ J}\)`

The dose in Gray is Joules per body mass

`\(= 0.02208 \text{ J} / 70 \text{ kg} = 3.15 * 10^(-4) \text{ Gray}\)`.

Answer 2

Q = 2.3 * 10⁻⁴ Gy

Step-by-step explanation:

The mass of the human body, M = 70 kg

Mass of potassium,
`m_(p) = 140 g`

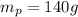
Atomic mass of potassium, MM = 39.1 g/mol

The half life of the isotope 40K,
`t_(1/2) = 1.3 * 10^(9)`

Number of moles of potassium,
`n = (m_(p) )/(MM)`

`n = (140)/(39.1)`

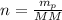
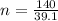
n = 3.58 mols

Let the number of atoms in the potassium be N

Number of mols of potassium,
`n = (N)/(6.02 * 10^(23) )`

`N = 6.02 * 10^(23) * n\\N = 6.02 * 10^(23) * 3.58\\N = 2.156 * 10^(24) atoms`

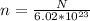
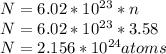
The 40K isotope has a natural abundance of 0.012% = 0.00012

That means the number of atoms originally in the 40K isotope is,
`N_(0) = 0.00012 * 2.156 * 10^(24)`

`N_(0) = 2.5875165 * 10^(20) atoms`

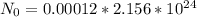
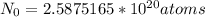
Number of atoms remaining after decay
`N_(f) = N - N_(0)`

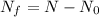
Decay constant,
`\lambda = (ln 2)/(t_(1/2) )`

`\lambda = (ln 2)/(1.3 * 10^9) \\\lambda = 533.19 * 10^(-12) yr^(-1)`

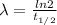
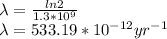
The question is asking for the yearly dose, i.e t = 1 yr

`N_(f) = N_(0) e^(- \lambda t) \\N_(f) = (2.5875165 * 10^(20) ) e^{- 533.19 * 10^(-12) *1}\\N_(f) = 2.587516499 * 10^(20)`

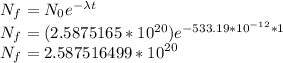
The number of 40K atoms decaying in 1 yr =
`N_(0) - N_(f) = (2.5875165 *10^(24) ) - (2.587516499 * 10^(20))`

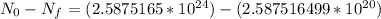
The number of 40K atoms decaying in 1 yr = 1 * 10¹¹ atoms

1 40K atom deposits 1.0MeV of energy into the body

1 * 10¹¹ atoms will deposit 1 * 10¹¹MeV of energy into the body = 10¹⁷ eV

1 eV = 1.6 * 10⁻¹⁹ Joules

10¹⁷ eV = (10¹⁷)*(1.6 * 10⁻¹⁹) Joules

10¹⁷ eV = 0.016 J

The amount of dose a typical body receives in Gy = 0.016/70

The amount of dose a typical body receives in Gy = 2.29 * 10⁻⁴