Question

What is the product?

StartFraction 4 k + 2 Over k squared minus 4 EndFraction times StartFraction k minus 2 Over 2 k + 1 EndFraction

Answer 1

`(4k + 2)/(k^(2)-4 )` ×
`(k-2)/(2k+1)` =
`(2)/(k + 2)`

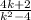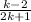Explanation:

`(4k + 2)/(k^(2)-4 )` ×
`(k-2)/(2k+1)`

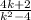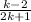
To solve the above, we need to follow the steps below;

4k+2 can be factorize, so that;

4k +2 = 2 (2k + 1)

k² - 4 can also be be expanded, so that;

k² - 4 = (k-2)(k+2)

Lets replace 4k +2 by 2 (2k + 1)

and

k² - 4 by (k-2)(k+2) in the expression given

`(4k + 2)/(k^(2)-4 )` ×
`(k-2)/(2k+1)`

`(2(2k+ 1))/((k-2)(k+2))` ×
`(k-2)/(2k+1)`

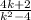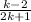
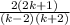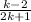
(2k+1) at the numerator will cancel-out (2k+1) at the denominator, also (k-2) at the numerator will cancel-out (k-2) at the denominator,

So our expression becomes;

`(2)/(k + 2)`

Therefore,
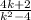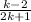`(4k + 2)/(k^(2)-4 )` ×
`(k-2)/(2k+1)` =
`(2)/(k + 2)`

Answer 2

The answer is D

Explanation: