Answer:
The 95% confidence interval for the measurements is [48.106, 53.494].
Explanation:
The average M of this sample is
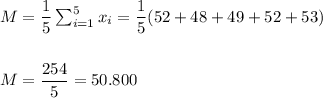
The standard deviation s of this sample is
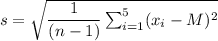
![s=\sqrt{(1)/(4)\cdot [(52-50.8)^2+(48-50.8)^2+(49-50.8)^2+(52-50.8)^2+(53-50.8)^2]}](https://img.qammunity.org/2021/formulas/mathematics/college/6n70qhrpu91e2mrjabehvkay6e0j2vquc4.png)
![s=\sqrt{(1)/(4)\cdot [(1.44)+(7.84)+(3.24)+(1.44)+(4.84)]}=\sqrt{(18.8)/(4)}=√(4.7)\\\\\\s=2.168](https://img.qammunity.org/2021/formulas/mathematics/college/becnudkxcy6y4lnt3rz9bpt94ft3owhrcb.png)
The degrees of freedom are
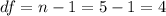
Then, the critical value of t for a 95% CI and 4 degrees of freedom is t=2.776.
The margin of error of the CI is:
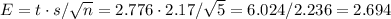
Then, the lower and upper bounds of the CI are:
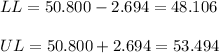
The 95% confidence interval for the measurements is [48.106, 53.494].