Question

A woman is 1.6 m tall and has a mass of 50 kg. She moves past an observer with the direction of the motion parallel to her height. The observer measures her relativistic momentum to have a magnitude of 2.4 × 1010 kg·m/s. What does the observer measure for her height?

Answer 1

L = 0.85m

Step-by-step explanation:

To find the height of the woman measured by the observer is necessary to use the Lorentz's contraction, given by the expression:

`L=L_o\sqrt{1-(v^2)/(c^2)}`

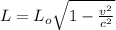
L: height of the woman measured by the observer

L_o: height of the woman in the her frame of reference

v: relative speed between the woman and the observer

c: speed of light = 3*10^ m/s

Lorentz's contraction can be used because the motion of the observer is parallel to the height of the woman.

Even though, it is necessary to compute the relative speed v. This is made by using the relativistic momentum formula:

`p=\frac{mv}{\sqrt{1-(v^2)/(c^2)}}\\\\p^2(c^2-v^2)=m^2v^2c^2\\\\v^2(m^2c^2+p^2)=p^2c^2\\\\v=(pc)/(√(m^2c^2+p^2))`

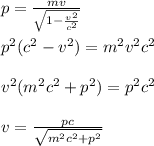
by replacing the values of p (2.4*10^10kgm/s) and m (50kg) you obtain:

`v=\frac{(2.4*10^(10)kgm/s)(3*10^8m/s)}{\sqrt{(50kg)^2(3*10^8m/s)^2+(2.4*10^(10)kgm/s)^2}}=2.54*10^(8)m/s`

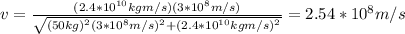
Finally, with the value of v you replace in the expression for L:

`L=(1.6m)\sqrt{1-((2.54*10^8m/s)^2)/((3*10^8m/s)^2)}=0.85m`

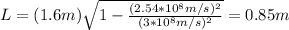
hence, the height of the woman measured by the observer is 0.85m