Question

A machine fills 50 kg bags with sand. The actual weight of sand in teh bags when the machine operates at its standard speed of 100 bages per hour has a normal distribution with a standard deviation of 0.75 kg. The mean of the distribution depends on the setting of the machine.

1. At what mean weight should the machine be set so that only 5% of the bags are underweight contain less than 50kg of sand?

Answer 1

The machine should be set at a mean weight of 51.23 kg.

Explanation:

Problems of normally distributed samples are solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

In this problem, we have that:

`\sigma = 0.75`

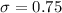
At what mean weight should the machine be set so that only 5% of the bags are underweight contain less than 50kg of sand?

We want 50 to be the 5th percentile.

So when X = 50, Z has a pvalue of 0.05. So when X = 50, Z = -1.645. We use this to find the mean weight
`\mu`

`Z = (X - \mu)/(\sigma)`

`-1.645 = (50 - \mu)/(0.75)`

`50 - \mu = -1.645*0.75`

`\mu = 51.23`

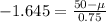
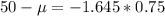
The machine should be set at a mean weight of 51.23 kg.