Question

When Carson runs the 400 meter dash, his finishing times are normally distributed with a mean of 65 seconds and a standard deviation of 2.5 seconds. Using the empirical rule, determine the interval of times that represents the middle 95% of his finishing times in the 400 meter race.

Answer 1

Given Information:

Mean time to finish 400 meter dash = μ = 65 seconds

Standard deviation to finish 400 meter dash = σ = 2.5 seconds

Confidence level = 95%

Required Information:

95% confidence interval = ?

Answer:

`CI = 60 \: to \: 70 \: seconds`

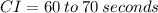
Step-by-step explanation:

In the normal distribution, the empirical rule states approximately 68% of all the data lie within 1 standard deviation from the mean, approximately 95% of all the data lie within 2 standard deviations from the mean and approximately 99.7% of all the data lie within 3 standard deviations from the mean.

The confidence interval for 95% confidence limit is given by

`CI = \mu \pm 2\sigma`

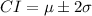
Since approximately 95% of all the data lie within 2 standard deviations from the mean. μ is the mean time Carson takes to finish 400 meter dash and σ is the standard deviation.

`CI = 65 \pm 2(2.5)`

`CI = 65 \pm 5`

`CI = 65 - 5 \: to \: 65 + 5`

`CI = 60 \: to \: 70 \: seconds`

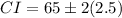
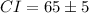
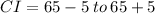
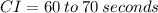
Therefore, the 95% confidence interval is between 60 to 70 seconds

What does it mean?

It means that we are 95% confident that the Carson's mean to finish 400 meter dash is within the interval of (60, 70).