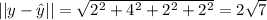Question

Find the distance from y to the subspace W of set of real numbers R Superscript 4ℝ4 spanned by Bold v 1v1 and Bold v 2v2​, given that the closest point to y in W is ModifyingAbove Bold y with caretyequals=[Start 4 By 1 Matrix 1st Row 1st Column 7 2nd Row 1st Column 5 3rd Row 1st Column negative 1 4st Row 1st Column 4 EndMatrix ]7 5 −1 4 .

Answer 1

`|| y - \hat{y} || = √(2^2 +4^2 +2^2 + 2^2) = 2 √(7)`

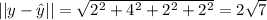
Explanation:

`y = \begin{pmatrix}-15 \\\ -1 \\\ 1 \\\ 2 \end{pmatrix}\\\\\\v_1 = \begin{pmatrix} 1 \\\ 1 \\\ -1 \\\ -2 \end{pmatrix}\\\\\\v_2 = \begin{pmatrix} 5 \\\ 1 \\\ 0 \\\ 3 \end{pmatrix}`

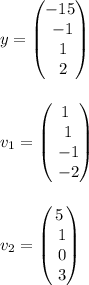
And according to the information given the closest point to
`y` in W is

`\hat{y} = \begin{pmatrix} -13 \\\ -5 \\\ 3 \\\ 0 \end{pmatrix}`

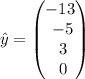
This is a problem when they give you more information than you actually need, by definition the distance from a vector to a subspace is given as the distance to the closest vector of the subspace, usually you have to find the vector using the Gram-Schmidt process and the orthogonal projection of the vector to the subspace, for this case you do not need any of that because the vector is given so all you find is the distance between
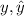`y,\hat{y}` which is

`|| y - \hat{y} || = √(2^2 +4^2 +2^2 + 2^2) = 2 √(7)`