Answer:
The percentage deviation is
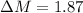 %
%
Step-by-step explanation:
From the question we are told that
The concentration is of the solution is

The true absorbance A = 0.7526
The percentage of transmittance due to stray light
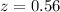 %
%
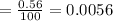
Generally Absorbance is mathematically represented as
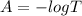
Where T is the percentage of true transmittance
Substituting value
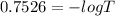
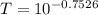
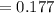
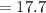 %
%
The Apparent absorbance is mathematically represented
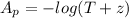
Substituting values
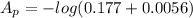
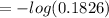
= 0.7385
The percentage by which apparent absorbance deviates from known absorbance is mathematically evaluated as
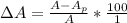
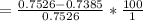
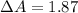 %
%
Since Absorbance varies directly with concentration the percentage deviation of the apparent concentration from know concentration is
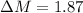 %
%