Question

t is known that the population variance equals 484. With a 0.95 probability, the sample size that needs to be taken if the desired margin of error is 5 or less is 25 74 189 75 None of the above answers is correct

Answer 1

The sample size needed to be taken is approximately 74.

Explanation:

To get alpha; we have 1 - 0.95 = 0.05

Therefore,
`z_{(a)/(2) } = (0.05)/(2) = 0.025`

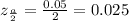
The we look up z* in a Standard Normal table where α = 0.025 area in each tail.

From the table, z* = 1.96

From the question variance is 484, then the standard deviation is 22

Standard deviation =
`√(Var(x)) = √(484) = 22`

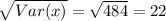
And margin of error is 5 or less

The formula for margin of error is given as:

margin of error =
`((z^(*)) (SD))/(√(n) )`

`5 = (1.96 * 22)/(√(n) ) \\5 = (43.12)/(√(n) ) \\5 * √(n) = 43.12\\(5)^(2) * (√(n) )^(2) = (43.12)^2\\25 * n = 1859.3344\\25n = 1859.3344\\(25n)/(25) = (1859.3344)/(25) \\n = 74.373376\\n = 74`

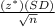
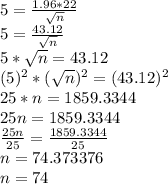
The approximate value of n is 74.