Question

We want to find the maximum and minimum values of f(x,y)=12x2+13y2 on the disk D: x2+y2≤1. What is the critical point in D? (x,y)=( , ) Now focus on the boundary of D, and solve for y2. Restricting f(x,y) to this boundary, we can express f(x,y) as a function of a single variable x. What is this function and its closed interval domain? f(x,y)=f(x)= where ≤x≤ What are the absolute maximum and minimum values of the function along the BOUNDARY of D? maximum value: minimum value: What are the absolute maximum and minimum values of f(x,y) over all of D? maximum value: minimum value:

Answer 1

Over the boundary: maximum:13, minimum:12

Over D: maximum:13, minimum:0

Explanation:

We are given that
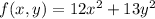`f(x,y) = 12x^2+13y^2` and D is the disc of radius one. Namely,
`x^2+y^2\leq 1`.

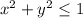First, we want to find a critical point of the function f. To do so, we want to find the values(x,y) such that

`\\abla f (x,y) =0`.

Recall that
`\\abla f (x,y) = ((\partial f)/(\partial x), (\partial f)/(\partial y))`.

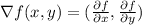So, let us calculate
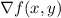`\\abla f (x,y)` (the detailed calculation of the derivatives is beyond the scope of the answer.

`(\partial f)/(\partial x) = 24x`

`(\partial f)/(\partial y) = 26y`

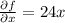
When equalling it to 0, we get that the critical point is (0,0), which is in our region D. Note that the function f is the sum of the square of two real numbers multiplied by some constants. Hence, the function f fulfills that
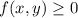`f(x,y)\geq 0`. Note that f(0,0)=0, so without further analysis we know that the point (0,0) is a minimum of f over D.

If we restrict to the boundary, we have the following equation
`x^2+y^2=1`. Main idea is to replace the value of one of the variables n the function f, so it becomes a function of a single variable. Then, we can find the critical values by using differential calculus:

Case 1:

Let us replace y. So, we have that
`y^2=1-x^2`. So,
`f(x,y) = 12x^2+13(1-x^2) = -x^2+13`.

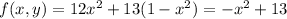So, we will find the derivative with respect to x and find the critical values. That is

`(df)/(dx) = -2x=0`

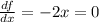
Which implies that x =0. Then,
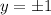`y =\pm 1`. So we have the following critical points (0,1), (0,-1). Notice that for both points, the value of f is f(0,1) = f(0,-1) = 13. If we calculate the second derivative, we have that at x=0

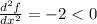`(d^2f)/(dx^2) = -2<0`. By the second derivative criteria, we know that this points are local maximums of the function f.

Case 2:

Let us replace x. So, we have that
`x^2=1-y^2`. So,
`f(x,y) = 12(1-y^2)+13y^2 = y^2+12`.

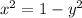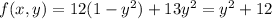So, we will find the derivative with respect to y and find the critical values. That is

`(df)/(dx) = 2y=0`

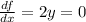
Which implies that y =0. Then,
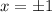`x =\pm 1`. So we have the following critical points (-1,0), (1,0). Notice that for both points, the value of f is f(1,0) = f(-1,0) = 12. If we calculate the second derivative, we have that at y=0

`(d^2f)/(dx^2) = 2>0`. By the second derivative criteria, we know that this points are local minimums of the function f.

So, over the boundary D, the maximum value of f is 13 and the minimum value is 12. Over all D, the maximum value of f is 13 and the minimum value is 0.