Question

We intend to estimate the average driving time of a group of commuters. From a previous study, we believe that the average time is 68 minutes with a standard deviation of 12 minutes. We want our 90 percent confidence interval to have a margin of error of no more than plus or minus 1 minutes. What is the smallest sample size that we should consider

Answer 1

Answer:392

Step-by-step explanation:

Answer 2

Answer: The smallest sample size would be 392.

Step-by-step explanation:

Since we have given that

Standard deviation = 12 minutes

Mean = 68 minutes

Margin of error =
`\pm 1`

At 90% confidence interval, z = 1.65

So, the smallest sample size that we should consider would be

`n=((z* \sigma)/(ME))^2\\\\n=((1.65* 12)/(1))^2\\\\n=(19.8)^2\\\\n=392.04\\\\n=392`

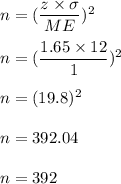
Hence, the smallest sample size would be 392.