Answer:
175.5 mm
Step-by-step explanation:
a hollow shaft of diameter ratio 3/8 is required to transmit 600kw at 110 rpm, the maximum torque being 20% greater than the mean. the shear stress is not to exceed 63 mpa and twist in a length of 3 meters not t exeed 1.4 degrees Calculate the minimum external diameter satisfying these conditions. G = 80 GPa
Let
D = external diameter of shaft
Given that:
d = internal diameter of the shaft = 3/8 × D = 0.375D,
Power (P) = 600 Kw, Speed (N) = 110 rpm, Shear stress (τ) = 63 MPa = 63 × 10⁶ Pa, Angle of twist (θ) = 1.4⁰, length (l) = 3 m, G = 80 GPa = 80 × 10⁹ Pa
The torque (T) is given by the equation:
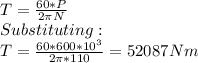
The maximum torque (
 ) = 1.2T = 1.2 × 52087 =62504 Nm
) = 1.2T = 1.2 × 52087 =62504 Nm
Using Torsion equation:
![(T)/(J) =(\tau)/(R)\\ J=(T.R)/(\tau) \\(\pi)/(32)[D^4-(0.375D)^4]=(62504*D)/(2(63*10^6)) \\D^3(0.9473)=0.00505\\D=0.1727m=172.7mm](https://img.qammunity.org/2021/formulas/engineering/college/cnwgk7welocq54i4zlyifpt18kbimwx94e.png)
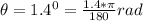
From the torsion equation:
![(T)/(J) =(G\theta )/(l)\\ J=(T.l)/(G\theta) \\(\pi)/(32)[D^4-(0.375D)^4]=(62504*3)/(84*10^9*(1.4*\pi)/(180) ) \\D=0.1755m=175.5mm](https://img.qammunity.org/2021/formulas/engineering/college/hf40f62zf5hqwfa6a725oamnr2b1d0obiv.png)
The conditions would be satisfied if the external diameter is 175.5 mm