Question

In a test of the effectiveness of garlic for lowering​ cholesterol, 36 subjects were treated with raw garlic. Cholesterol levels were measured before and after the treatment. The changes​ (before minus​ after) in their levels of LDL cholesterol​ (in mg/dL) have a mean of 0.9 and a standard deviation of 15.8. Use a 0.01 significance level to test the claim that with garlic​ treatment, the mean change in LDL cholesterol is greater than 0. What do the results suggest about the effectiveness of the garlic​ treatment? Assume that a simple random sample has been selected. Identify the null and alternative​ hypotheses, test​ statistic, P-value, and state the final conclusion that addresses the original claim.

Answer 1

Answer: We do not sufficient evidence that mean is greater than 0.

Explanation:

Since we have given that

n = 36

mean = 0.9

Standard deviation = 15.8

at 0.01 level of significance,

Hypothesis would be:

`H_0:\mu =0\\\\H_1=\mu\\eq 0`

Standard error of mean would be :

`(\sigma)/(√(n))=(15.8)/(√(36))=(15.8)/(6)=2.63`

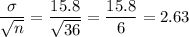
statistic value would be :

`t=\frac{\bar{x}-\mu}{(\sigma)/(√(n))}\\\\t=(0.9-0)/(2.63)\\\\t=(0.9)/(2.63)\\\\t=0.342`

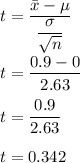
Degree of freedom = df = 36-1=35

So, p value = 2.4377

Since 2.4377 > 0.342, we will not reject null hypothesis.

Hence, We do not sufficient evidence that mean is greater than 0.