Answer:
The obsrved frquencies are
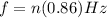 n = 1,2,3,...,n.
n = 1,2,3,...,n.
Step-by-step explanation:
From the question we are told that
The speed of the wave is v = 0.740 m/s
The distance from the post is
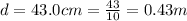
Generally frequency is mathematically represented as
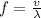
so for the first frequency which the the fundamental frequency(first harmonic frequency) the clothesline(string) would form only one loop and hence the length between the vertical post and throcky hands is mathematically represented as
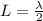
=>

So
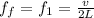
Substituting values
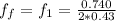

For the second harmonic frequency i.e is when the clothesline(string) forms two loops the length is mathematically represented as
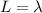
So the second harmonic frequency is
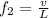
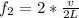
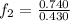
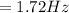
For the third harmonic frequency i.e when the clothesline(string) forms three loops the length is mathematically represented as
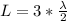
So the wavelength is
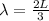
And the third harmonic frequency is mathematically evaluated as
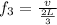
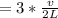
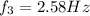
Looking at the above calculation we can conclude that the harmonic frequency of a vibrating string(clothesline) can be mathematically represented as an integer multiple of the fundamental frequency
i.e
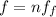
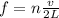
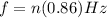
Where n denotes integer values i.e n = 1,2,3,....,n.
Note : The length that exist between two nodes which are successive is equivalent to half of the wavelength so when one loop is form the number of nodes would be 2 and on anti-node