Question

In a skating stunt known as "crack-the-whip", a number of skaters hold hands and form a straight line. They try to skate so that the line rotates about the skater at one end, who acts as the pivot. The skater farthest out has a mass of 83.7 kg and is 6.11 m from the pivot. He is skating at a speed of 6.83 m/s. Determine the magnitude of the centripetal force that acts on him.

Answer 1

639.03N

Step-by-step explanation:

you can assume that the centripetal force over the last skater is only due to the tension from the other skaters. Thus, you can use the formla for the centripetal force:

`F_c=(mv^2)/(r)`

m: mass of the skater = 83.7kg

v: velocity of the skater = 6.83m/s

r: distance to the pivot = 6.11m

By replacing you obtain:

`F_c=((83.7kg)(6.83m/s)^2)/(6.11m)=639.03N`

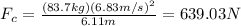
hence, the centripetal force is 639.03N