Question

This is due, in part, to damage from the first episode. The performance of a new drug designed to prevent a second episode is to be tested for its effectiveness in preventing a second episode. In order to do this two groups of people suffering a first episode are selected. There are 163 people in the first group and this group will be administered the new drug. There are 160 people in the second group and this group wil be administered a placebo. After one year, 13% of the first group has a second episode and 14% of the second group has a second episode. Select a 90% confidence interval for the difference in true proportion of the two groups.

Answer 1

Δπ Min = -0.0709

Δπ Max = -0.0535

Explanation:

Here we have

`z=\frac{(\hat{p_(1)}-\hat{p_(2)})-(\mu_(1)-\mu _(2) )}{\sqrt{\frac{\hat{p_(1)}(1-\hat{p_(1)}) }{n_(1)}-\frac{\hat{p_(2)}(1-\hat{p_(2)})}{n_(2)}}}`

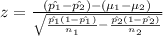
Where:

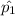`{\hat{p_(1)}` = 13% = 0.13

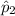`\hat p_(2)` = 14% = 0.14

n₁ = 163

n₂ = 160

Therefore, we have;

`z=\frac{(\hat{p_(1)}-\hat{p_(2)})}{\sqrt{\frac{\hat{p_(1)}(1-\hat{p_(1)}) }{n_(1)}-\frac{\hat{p_(2)}(1-\hat{p_(2)})}{n_(2)}}}`

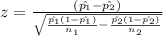
Plugging the values gives

z = -0.263

CI 90% = critical z =
`\pm`1.644

The minimum difference in true proportion = -0.0709

The maximum difference in true proportion = 0.0535.