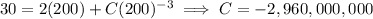Salt flows in at a rate of
(2 g/L) * (4 L/min) = 8 g/min
and out at a rate of
(B/(200 + t) g/L) * (3 L/min) = 3B/(200 + t) g/min
where B is the amount of salt in the tank at time t.
Then the net rate at which B changes is governed by the ODE,
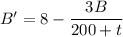
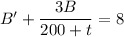
Multipy both sides by
 :
:
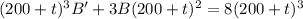
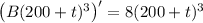
Integrate both sides:

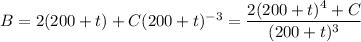
The tank starts with 30 g of salt, so B(0) = 30, which gives