Answer:
The calculated 't' value = 0.9822
The degrees of freedom = n-1 =40-1 =39
The calculated 't' value = 0.9822< 2.0192 at 95% level of significance with 39 degrees of freedom.
Null hypothesis is accepted at 95% level of significance.
There is evidence that bumblebee bats weight less on average than he claims.
Explanation:
Step:- (1)
Given the mean weight of 40 randomly selected bumblebee bats is 1.659 grams, with a standard deviation of 0.264 grams.
Given sample size 'n' = 40
mean of the sample x⁻ = 1.659 grams
Standard deviation of the sample 'S' = 0.264 grams
Dr. Clifford Jones claims that the mean weight of bumblebee bats is 1.7 grams.
Population mean 'μ' = 1.7grams
Step:-(2)
Null hypothesis: H₀: There is evidence that bumblebee bats weight less on average than he claims.
Alternative hypothesis : H₁ :- There is no evidence that bumblebee bats weight less on average than he claims.
The degrees of freedom γ= n-1 =40-1 =39
we will choose level of significance = 0.95 0r 0.05
Step:-(3)
The test statistic
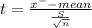
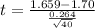
t = -0.9822
|t|=|-0.9822|
t = 0.9822
The calculated 't' value = 0.9822
The tabulated value t = 2.0192 at 95% level of significance.
The calculated 't' value = 0.9822 < 2.024 at 95% level of significance with 39 degrees of freedom.
Null hypothesis is accepted at 95% level of significance.
Conclusion:-
There is evidence that bumblebee bats weight less on average than he claims.