To solve this problem we will apply the concepts related to continuity and later to Bernoulli's principle. With the continuity equation we will relate the diameters of the two measurements to find the speed. Later with the speed we will proceed to replace it in the Bernoulli equations to find the pressure.
By continuity equation

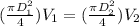
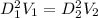
With the relation of diameters we have
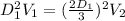

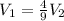
Replacing the value of the Volume
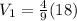
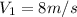
By Bernoulli's equation
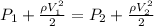
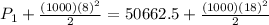

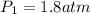
Therefore the water pressure in the wide section is 1.8atm