Question

32) In one region, the September energy consumption levels for single-family homes are found to be normally distributed with a mean of 990 kWh and a standard deviation of 198 kWh. For a randomly selected home, find the probability that the September energy consumption level is between 1100 kWh and 1250 kWh.

Answer 1

0.1946 is the probability that the September energy consumption level is between 1100 kWh and 1250 kWh.

Explanation:

We are given the following information in the question:

Mean, μ = 990 kWh

Standard Deviation, σ = 198 kWh

We are given that the distribution of energy consumption levels is a bell shaped distribution that is a normal distribution.

Formula:

`z_(score) = \displaystyle(x-\mu)/(\sigma)`

P(September energy consumption level is between 1100 kWh and 1250 kWh)

`P(1100 \leq x \leq 1250)\\\\ = P(\displaystyle(1100 - 990)/(198) \leq z \leq \displaystyle(1250 -990)/(198)) \\\\= P(0.5556 \leq z \leq 1.3131)\\\\= P(z \leq 1.3131) - P(z < 0.5556)\\\\= 0.9054- 0.7108= 0.1946`

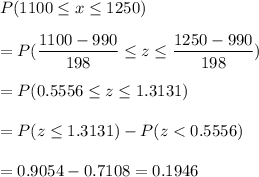
0.1946 is the probability that the September energy consumption level is between 1100 kWh and 1250 kWh.