Answer:
28 pints, 112 pints
Explanation:
GIVEN: The Royal Fruit Company produces two types of fruit drinks. The first type is
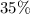 pure fruit juice, and the second type is
pure fruit juice, and the second type is
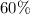 pure fruit juice. The company is attempting to produce a fruit drink that contains
pure fruit juice. The company is attempting to produce a fruit drink that contains
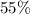 pure fruit juice.
pure fruit juice.
TO FIND: How many pints of each of the two existing types of drink must be used to make
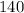 pints of a mixture that is
pints of a mixture that is
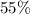 pure fruit juice.
pure fruit juice.
SOLUTION:
Let the quantity of first type of juice be

Quantity of second type of juice
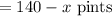
Concentration of pure juice in final mixture
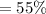
Now,
The concentration of pure juice in final mixture is sum of concentrations of pure juice in first and second type of juice

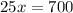
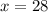
Quantity of first type of juice
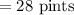
Quantity of second type of juice

Hence quantity of first and second type of juice is 28 pints and 112 pints respectively.