Answer:
Cathy is asked to find the length of AC. She could use the Pythagorean Theorem and AC^2 = 3^2 + 2^2. What other formula could she use?
A) (0 + 3)^2 - (1 + 3)^2
B) (0 + 1)^2 - (3 + 3)^2
C) (0 - 3)^2 + (1 - 3)^2
D) (0 - 1)^2 + (3 - 3)^2
Option C is the right choice.
Explanation:
Given:
Cathy have used Pythagoras formula to find the hypotenuse.
Hypotenuse of the right angled triangle = AC
We know that:
In right angled triangle:
Hypotenuse square (h)^2 = Square of one side (p)^ + Square of another sides (b)^
⇒
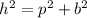
In Cathy's calculation:
⇒
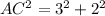
⇒
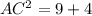
⇒
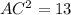
We have to look for another equation.
Lets see the options individually.
A.

B.

C.
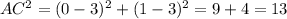
D.
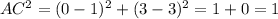
So,
The other formula Cathy can use is, C i.e. (0 - 3)^2 + (1 - 3)^2 .
Option C is the right choice.