Answer:
Explanation:
Hello!
The objective of this exercise is to compare the proportion of defective parts produced by machine 1 and machine 2.
The parameter of study is the difference between the population proportion of defective parts produced by machine 1 and the population proportion of defective parts produced by machine 2, symbolically: p₁ - p₂
The hypotheses are:
H₀: p₁ - p₂ ≤ 0
H₁: p₁ - p₂ > 0
α: 0.03
This hypothesis test is one-tailed to the right, which means that you will reject the null hypothesis with high values of the statistic.
To test the difference of proportions you have to use a standard normal distribution, the critical value will be:
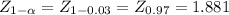
The decision rule using the critical value approach is:
If
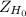 ≥ 1.881, the decision is to reject the null hypothesis.
≥ 1.881, the decision is to reject the null hypothesis.
If
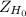 < 1.881, the decision is to not reject the null hypothesis.
< 1.881, the decision is to not reject the null hypothesis.
Considering the calculated
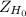 < 1.881, the decision is to not reject the null hypothesis. Using a significance level of 3%, you can conclude that the difference between the population proportion of defective plastic parts produced by machine 1 and the population proportion of defective plastic parts produced by machine 2 is at most zero.
< 1.881, the decision is to not reject the null hypothesis. Using a significance level of 3%, you can conclude that the difference between the population proportion of defective plastic parts produced by machine 1 and the population proportion of defective plastic parts produced by machine 2 is at most zero.
I hope this helps!