Question

The data show systolic and diastolic blood pressure of certain people. Find the regression​ equation, letting the systolic reading be the independent​ (x) variable. If one of these people has a systolic blood pressure of 113 mm​ Hg, what is the best predicted diastolic blood​ pressure?

Systolic| 150 129 142 112 134 122 126 120Diastolic| 88 96 106 80 98 63 95 64

Answer 1

The predicted diastolic blood​ pressure of people with 113 mm Hg systolic blood pressure is 74 mm Hg.

Explanation:

The general form of a regression equation is:

`y=\alpha +\beta x`

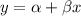
Here,

y = dependent variable

x = independent variable

α = intercept

β = slope

The formula to compute the slope and intercept are:

`\begin{aligned} \alpha &= \frac{\sum{Y} \cdot \sum{X^2} - \sum{X} \cdot \sum{XY} }{n \cdot \sum{X^2} - \left(\sum{X}\right)^2} \\\\\beta &= \frac{ n \cdot \sum{XY} - \sum{X} \cdot \sum{Y}}{n \cdot \sum{X^2} - \left(\sum{X}\right)^2} \end{aligned}`

The value of
`\sum X,\ \sum Y,\ \sum XY\ and\ \sum X^(2)` as compute in the table attached below.

Compute the value of α and β as follows:

`\begin{aligned} \alpha &= \frac{\sum{Y} \cdot \sum{X^2} - \sum{X} \cdot \sum{XY} }{n \cdot \sum{X^2} - \left(\sum{X}\right)^2} = ( 690 \cdot 134965 - 1035 \cdot 90064)/( 8 \cdot 134965 - 1035^2) \approx -10.64 \\ \\\beta &= \frac{ n \cdot \sum{XY} - \sum{X} \cdot \sum{Y}}{n \cdot \sum{X^2} - \left(\sum{X}\right)^2} = ( 8 \cdot 90064 - 1035 \cdot 690 )/( 8 \cdot 134965 - \left( 1035 \right)^2) \approx 0.749\end{aligned}`

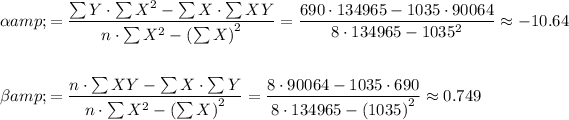
Thus, the regression equation of diastolic blood pressure based on systolic blood pressure is:

`y=-10.64+0.749x`

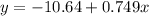
Compute the value of y for x = 113 as follows:

`y=-10.64+0.749x`

`=-10.64+0.749* 113\\=-10.64+84.637\\=73.997\\\approx 74`

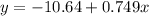
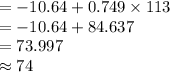
Thus, the predicted diastolic blood​ pressure of people with 113 mm Hg systolic blood pressure is 74 mm Hg.