Question

Aaron creates a stacked cake comprised of three cylindrical sections and plans to cover the top of each section with a different color icing. Each lower section is a total of two inches wider than the section above it. If the yellow top of the upper section has an area of 12.56 square inches, what is the approximate area of the green visible portion of the bottom section which he will need to cover with icing?

Answer 1

Option C

Explanation:

Complete question is

Each lower section is a total of two inches wider than the section above it. If the yellow top of the upper section has an area of 12.56 square inches, what is the approximate area of the green visible portion of the bottom section which he will need to cover with icing?

A. 13 square inches

B. 88 square inches

C. 49 square inches

D. 22 square inches

Solution -

Given

Top of each cylindrical section is covered with a different color icing

Radius of each color icing is two inches less than the section below it.

Let the radius of the icing be "r"

Then the surface area of the icing will be
`\pi r^2`

Now area of the yellow icing is equal to
`12.56` square inches

Thus,

`\pi r^(2) = 12.56`

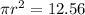
On solving the above equation for r, we get -

`r^2 = (12.56)/(3.14) \\r^2 = 4\\r = √(4) \\`

r = 2 inches

Now radius of the section below the icing is equal to

`r + 2\\= 2+ 2\\`

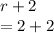
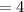`= 4` inches

Area of the section below the icing

`= \pi r^2\\= 3.14 * 4^2\\= 50.24`

Thus, option C is correct