Question

A ball is thrown from an initial height of 3 feet with an initial upward velocity of 44 ft/s. The ball's height h (in feet) after seconds is given by the following.

h = 3+44t-16t^2

Find all values of t which the balls height is 23 feet.

Answer 1

t = 2.175 s (3 dp)

t = 0.575 s (3 dp)

Explanation:

Given equation:
`h=3+44t-16t^2`

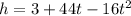
To find all values of t for which the ball's height is 23 ft, substitute h = 20 into the equation and solve for t:

`\implies h=23`

`\implies 3+44t-16t^2=23`

`\implies 16t^2-44t-3+23=0`

`\implies 16t^2-44t+20=0`

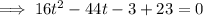
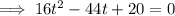
Factor out common term 4:

`\implies 4(4t^2-11t+5)=0`

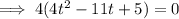
Divide both sides by 4:

`\implies 4t^2-11t+5=0`

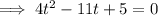
Quadratic formula

`x=(-b \pm √(b^2-4ac) )/(2a)\quad\textsf{when}\:ax^2+bx+c=0`

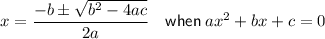
Use the quadratic formula to solve for t:

`\implies t=(-(-11) \pm √((-11)^2-4(4)(5)) )/(2(4))`

`\implies t=(11 \pm √(41))/(8)`

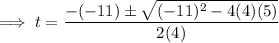
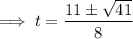
Therefore,

• t = 2.175 s (3 dp)
• t = 0.575 s (3 dp)

Answer 2

See below ↓↓

Explanation:

Given function

• h = 3 + 44t - 16t²

We need to calculate the possible values of t at h = 23 feet.

Subsituting h = 23,

• 23 = 3 + 44t - 16t²
• 16t² - 44t + 20 = 0
• Divide throughout by 4 as it is a common factor
• 4t² - 11t + 5 = 0

Solving for 't' using quadratic formula

• t = 11 ± √121 - 4(4)(5) / 8
• t = 11 ± √41 / 8

Solutions

1. t = 11 + 6.4 / 8 = 17.4/8 = 2.175 seconds
2. t = 11 - 6.4 / 8 = 4.6/8 = 0.575 seconds