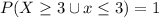Question

(a) Identify and correct any mistakes in the probability distribution: There may be more than one way to make corrections. (b) Find the expected value of the amended distribution. (c) Find the probability that x is at most 3. (d) Find the probability that x is at least 3. e) Find the probability that x is at least 3 or at most 3. X P(x) xP(x) -1 0.15 -0.15 0 -0.25 0.25 2 0.30 0.60 3 0.11 0.14 4 0 .29 4.29

Answer 1

a) For this case we have the following probability distribution given:

X -1 0 2 3 4

P(X) 0.15 -0.25 0.30 0.11 0.29

XP(X) -0.15 0.25 0.60 0.14 4.29

For any probability distribution we need to satisfy two conditions:

`p(X_i) \geq 0 , i =1,2,...,n`

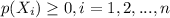
And then the value os -0.25 is not correct. The second condition is:

`\sum_(i=1)^n P(X_i) =1`

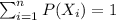
And for this case s we use 0.15+0.25+0.30+0.11+0.29 = 1.1>1 so then we need to fix this condition too. The other problems are related to the column xP(X) are incorrect. Here is an example of a probability distribution purposed

b) X -1 0 2 3 4

P(X) 0.15 0.25 0.30 0.12 0.29

And for this case the expected value would be:

`E(X) = \sum_(i=1)^n X_i P(X_i)`

`E(X) = -1*0.15 +0*0.25 +2*0.30 +3*0.12 +4*0.29 = 1.97`

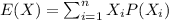
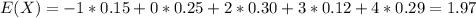
c)
`P(x \leq 3) = P(X=-1)+P(X=0) +P(X=2) +P(X=3) = 0.15+0.25+0.30+0.12= 0.82`

d) P(X \geq 3) = P(X=3) +P(X=4)= 0.12+0.29=0.41[/tex]

e) For this case we can use the total rule of probability and we got:

`P(X \geq 3 \cup x\leq 3)=1`

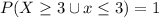
Explanation:

Part a

For this case we have the following probability distribution given:

X -1 0 2 3 4

P(X) 0.15 -0.25 0.30 0.11 0.29

XP(X) -0.15 0.25 0.60 0.14 4.29

For any probability distribution we need to satisfy two conditions:

`p(X_i) \geq 0 , i =1,2,...,n`

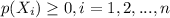
And then the value os -0.25 is not correct. The second condition is:

`\sum_(i=1)^n P(X_i) =1`

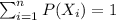
And for this case s we use 0.15+0.25+0.30+0.11+0.29 = 1.1>1 so then we need to fix this condition too. The other problems are related to the column xP(X) are incorrect. Here is an example of a probability distribution purposed

Part b

X -1 0 2 3 4

P(X) 0.15 0.25 0.30 0.12 0.29

And for this case the expected value would be:

`E(X) = \sum_(i=1)^n X_i P(X_i)`

`E(X) = -1*0.15 +0*0.25 +2*0.30 +3*0.12 +4*0.29 = 1.97`

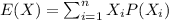
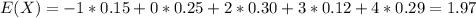
Part c

`P(x \leq 3) = P(X=-1)+P(X=0) +P(X=2) +P(X=3) = 0.15+0.25+0.30+0.12= 0.82`

Part d

P(X \geq 3) = P(X=3) +P(X=4)= 0.12+0.29=0.41[/tex]

Part e

For this case we can use the total rule of probability and we got:

`P(X \geq 3 \cup x\leq 3)=1`