Question

A closed thermodynamic cycle consists of two isobaric and two isochoric processes. The processes operate between 0.43 MPa and 4.3 MPa and 0.28 m3 and 2.9 m3. Determine the total work (with appropriate sign) for one complete cycle, when the cycle operates in the clockwise direction. Provide your result in MJ.

Answer 1

Step-by-step explanation:

For first isobaric process (expansion with constant pressure):

`W_(1)=P_(1)(V_(2)-V_(1))=(0.43* 10^(6))(2.9-0.28)=1.1266 * 10^(6) J=1.1266 MJ(+) (Expansion)`

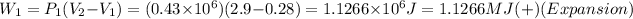
Now, for the first isochoric:
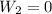`W_(2)=0`, and
`Q_(2)=\Delta U=U_(3)-U_(2)`

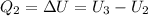
For the second isobaric process (compression with constant pressure):

`W_(3)=P_(2)(V_(1)-V_(2))=(4.3* 10^(6))(0.28-2.9) =-11.266* 10^(6)J=-11.266 MJ (Compression)`

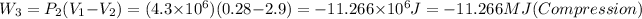
For the last isochoric:
`W_(4)=0, Q_(4)=\Delta U=U_(4)-U_(3)`

So, the total work per cycle:
`W=W_(1)+W_(2)+W_(3)+W_(4)=1.1266+0-11.266+0=-10.1394 MJ`