Given:
The height of the given trapezoid = 6 in
The area of the trapezoid = 72 in²
Also given, one base of the trapezoid is 6 inches longer than the other base
To find the lengths of the bases.
Formula
The area of the trapezoid is

where, h be the height of the trapezoid
 be the shorter base
be the shorter base
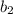 be the longer base
be the longer base
As per the given problem,
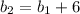
Now,
Putting, A=72,
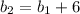 and h=6 we get,
and h=6 we get,
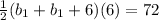
or,
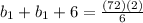
or,

or,
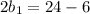
or,

or,
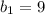
So,
The shorter base is 9 in and the other base is = (6+9) = 15 in
Hence,
One base is 9 inches for one of the bases and 15 inches for the other base.