Question

Suppose light from a 632.8 nm helium-neon laser shines through a diffraction grating ruled at 520 lines/mm. How many bright lines are formed on a screen a distance away?

Answer 1

1 bright fringe every 33 cm.

Step-by-step explanation:

The formula to calculate the position of the m-th order brigh line (constructive interference) produced by diffraction of light through a diffraction grating is:

`y=(m\lambda D)/(d)`

where

m is the order of the maximum

`\lambda` is the wavelength of the light

D is the distance of the screen

d is the separation between two adjacent slit

Here we have:

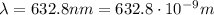`\lambda=632.8 nm = 632.8\cdot 10^(-9) m` is the wavelength of the light

D = 1 m is the distance of the screen (not given in the problem, so we assume it to be 1 meter)

`n=520 lines/mm` is the number of lines per mm, so the spacing between two lines is

`d=(1)/(n)=(1)/(520)=1.92\cdot 10^(-3) mm = 1.92\cdot 10^(-6) m`

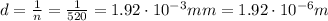
Therefore, substituting m = 1, we find:

`y=((632.8\cdot 10^(-9))(1))/(1.92\cdot 10^(-6))=0.330 m`

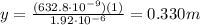
So, on the distant screen, there is 1 bright fringe every 33 cm.