Question

You have a wire that is 74 cm long. You wish to cut it into two pieces. One piece will be bent into the shape of a square. The other piece will be bent into the shape of a circle. Let A represent the total area of the square and the circle. What is the circumference of the circle when A is a minimum

Answer 1

32.55 cm

Explanation:

Let x = the length of wire that becomes a circle.

Then 74 - x = the length of wire that becomes a square

Circumference of circle + perimeter of square = 74

1. Expression for the side of the square

P = 4s = 74 - x

s = ¼(74 - x)

2. Expression for the radius of the circle

C = 2πr = x

r = x/(2π)

3. Expression for the total area

`\begin{array}{rcl}\text{Total area} & = &\text{area of circle+ area of square}\\A & = & \pi r^(2) + s^(2)\\& = &\pi \left((x)/(2 \pi)\right)^(2) + \left ((1)/(4)(74 - x)\right)^(2)\\\\ & = & (x^(2))/(4 \pi) + (1)/(16)(5476 - 148x + x^(2))\\\\ & = & 0.07958x^(2) + 342.25 - 9.25x + 0.0625x^(2)\\A & = & 0.1421x^(2) -9.25x + 342.25 \\ \end{array}`

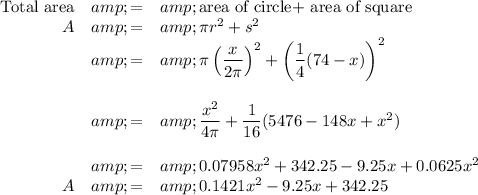
This is the equation of a parabola.

In standard form,

ƒ(x) = 0.1421x² -9.25x + 342.24

a = 0.1421; b = -9.25; c = 342.24

The parabola opens upwards, because a > 0. Therefore, the vertex is a minimum.

The vertex of a parabola occurs at

x = -b/(2a) = 9.25/(2 × 0.1421) = 9.25/(0.2842) = 32.55

The circumference of the circle is 32.55 cm.

The graph below shows that the area of the circle is a minimum when x = 32.55 cm