Question

g What is the magnetic potential energy stored in a cylindrical volume of height hcylinhcylin = 50 mmmm and radius RcylinRcylin = 24 mmmm that symmetrically surrounds an infinitely long wire that has radius RwireRwire = 2.1 mmmm and carries current III = 4.9 AA ? The volume in which the energy should be calculated does not contain the wire.

Answer 1

`2.9\cdot 10^(-7) J`

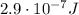
Step-by-step explanation:

The energy density associated to a magnetic field is:

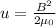`u=(B^2)/(2\mu_0)` (1)

where

B is the strength of the magnetic field

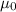`\mu_0` is the vacuum permeability

The magnetic field produced by a current-carrying wire is

`B=(\mu_0 I)/(2\pi r)`

where

I is the current in the wire

r is the distance from the wire at which the field is calculated

Substituting into (1),

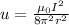`u=(\mu_0 I^2)/(8\pi^2 r^2)` (2)

Since this is the energy density, the total energy stored in a certain element of volume
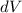`dV` will be

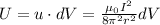`U=u\cdot dV=(\mu_0I^2)/(8\pi^2 r^2)dV` (3)

Here the field strength changes as we move farther from the wire radially, so we can write dV as

`dV=2\pi h r dr`

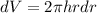
where

h is the height of the cylinder

r is the distance from the wire

So eq(3) becomes:

`dU=(\mu_0I^2)/(8\pi^2 r^2) \cdot 2 \pi h r dr = (\mu_0 I^2 h)/(4\pi)(1)/(r)dr`

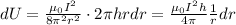
Now we have to integrate this expression to find the total energy stored in the cylindrical volume. We have:

h = 50 mm = 0.050 m is the height of the cylinder

I = 4.9 A is the current in the wire

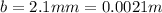`b=2.1 mm = 0.0021 m` is the internal radius of the cylinder (the radius of the wire)

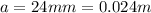`a=24 mm=0.024 m` is the external radius of the cylinder

So,

`U=\int\limits^a_b {dU} =(\mu_0 I^2 h)/(4\pi) \int\limits^(0.024)_(0.0021) (1)/(r)dr = (\mu_0 I^2 h)/(4\pi) [ln(a)-ln(b)]=\\=((4\pi \cdot 10^(-7))(4.9)^2(0.050))/(4\pi)[ln(0.024)-ln(0.0021)]=2.9\cdot 10^(-7) J`