Question

The number of students in an elementary school t years after 2002 is given by s(t) = 100 ln(t + 5) students. The yearly cost to educate one student t years after 2002 can be modeled as c(t) = 1500(1.05t) dollars per student. (a) What are the input units of the function f(t) = s(t) · c(t)?

Answer 1

The input units of the function f(t) are students multiplied by dollars per student.

The function
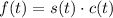`\( f(t) = s(t) \cdot c(t) \)` represents the total cost to educate all the students in the elementary school t years after 2002. To determine the input units of this function, we need to analyze the units of its individual components.

s(t) is the number of students at time t. Its unit is "students."

c(t) is the cost to educate one student at time t. Its unit is "dollars per student."

When you multiply s(t) by c(t), the units combine as follows:

`\[ f(t) = s(t) \cdot c(t) = \text{`

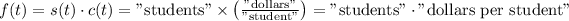
Therefore, the input units of the function f(t) are students multiplied by dollars per student.