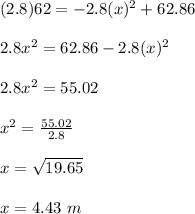Answer:
v = 5.69 m/s
x = 4.43 m
Step-by-step explanation:
Mass of the body m = 2.5 kg
force acting on the body
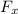 = - 7 x N
= - 7 x N
From the Newton's second Law;
 ; Then:
; Then:
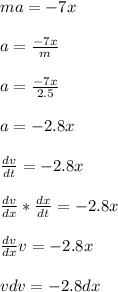
Integrating on both sides ; we have :

where; k is the integral constant ;
At x = 2.6 m speed is 8.5 m/s
Then;
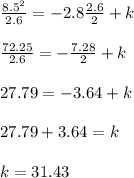
However:

a)
At x = 3.3 m; speed of the object

b)
speed of the body is 2.8 m/s; then