Question

A swim school is expanding and needs to take out a one-year, $55,000 loan for a new lap pool. Bank A says it can give the swim school a 25% annual interest rate compounded once. This is represented with the equation A=55,000(1.25)t. A = 55,000 ( 1.25 ) t . Bank B says it can offer the swim school a semi-annual interest rate that will be compounded twice. In order for the loan at Bank B to cost the swim school the same amount, what APR would Bank B need to offer?

Answer 1

Therefore the bank need to offer 23.6% annual interest rate compounded twice.

Explanation:

Compound interest formula:

`A=P(1+\frac rn)^(nt)`

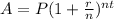
A=Amount

P=Principal

r=rate of interest

n= Number of times interest is compounded per year.

t= time

Bank A

P=$55,000, r=25% = 0.25, n=1, t=t

The amount that the school have to pay after t year is

`A_1=55,000(1+0.25)^t`

`=55000(1.25)^t`

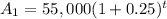
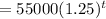
Bank B

P=$55,000, r=?, n=2, t=t

The amount that the school have to pay after t year is

`A_2=55,000(1+\frac r2)^(2t)`

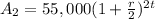
Since the amount for both banks are same.

i.e

`A_1=A_2`

`\Rightarrow 55000(1.25)^t=55000(1+\frac r2)^(2t)`

`\Rightarrow (1.25)^t=(1+\frac r2)^(2t)`

`\Rightarrow (1.25)=(1+\frac r2)^(2)`

`\Rightarrow (1+\frac r2)=√(1.25)`

`\Rightarrow (1+\frac r2)=1.118`

`\Rightarrow \frac r2=1.118-1`

`\Rightarrow \frac r2=0.118`

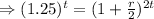
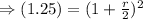
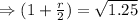
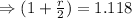
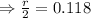
⇒r=0.118×2

⇒r = 0.236

⇒r =23.6%

Therefore the bank need to offer 23.6% annual interest rate compounded twice.