Answer:
We conclude that the the manufacturer's advertising campaign is not correct.
P-value of test = 0.067.
Step-by-step explanation:
We are given that an independent testing service road-tested 36 of the automobiles. The sample showed an average of 51.5 miles per gallon. The population standard deviation is 6 miles per gallon.
We have to conduct a test to determine whether or not the manufacturer's advertising campaign is legitimate.
Let
 = population average gasoline of new small cars in highway driving.
= population average gasoline of new small cars in highway driving.
SO, Null Hypothesis,
 :
:

 50 miles per gallon {means that the manufacturer's advertising campaign is not correct}
50 miles per gallon {means that the manufacturer's advertising campaign is not correct}
Alternate Hypothesis,
 :
:
 > 50 miles per gallon {means that the manufacturer's advertising campaign is correct}
> 50 miles per gallon {means that the manufacturer's advertising campaign is correct}
The test statistics that will be used here is One-sample z test statistics as we know about the population standard deviation;
T.S. =
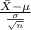 ~ N(0,1)
~ N(0,1)
where,
 = sample average gasoline = 51.5 miles per gallon
= sample average gasoline = 51.5 miles per gallon
 = population standard deviation = 6 miles per gallon
= population standard deviation = 6 miles per gallon
n = sample of automobiles = 36
So, test statistics =

= 1.50
Now at 0.05 significance level, the z table gives critical value of 1.6449 for right-tailed test. Since our test statistics is less than the critical values of z as 1.50 < 1.6449, so we have insufficient evidence to reject our null hypothesis as it will not fall in the rejection region.
Also, P-value is given by the following formula;
P-value = P(Z > 1.50) = 1 - P(Z
 1.50)
1.50)
= 1 - 0.93319 = 0.067 or 6.7%
Therefore, we conclude that the the manufacturer's advertising campaign is not correct as its new small cars average less than or equal to 50 miles per gallon in highway driving.