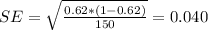Question

In a recent survey of 150 teenagers, 93 stated that they always wear their seatbelt when they travel in a car. Assuming the distribution is approximately normal, find the point estimate and standard error for the proportion of teenagers that always wear a seatbelt when traveling in a car. Round your answers to three decimal places, as needed.

Answer 1

p'=.62

Op'=.04

Explanation:

To find p' divide 93 by 150:

93/150=.62

To find Op' put p' into the equation:
`\sqrt{.62(1-.62)/150` which is .0396 rounded to .04

Answer 2

`\hat p \sim N (p ,\sqrt{(p*(1-p))/(n)}`

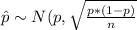
The estimated standard error is given by:

`SE= \sqrt{(0.62*(1-0.62))/(150)}=0.040`

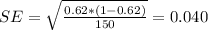
Explanation:

For this case we have the following data:

n =150 represent the sample size selected

x = 93 people stated that they always wear their seatbelt when they travel in a car

For this case the the proportion estimated is :

`\hat p = (93)/(150)= 0.62`

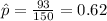
We can can check if we can use the normal approximation :

`np =150*0.62= 93>10`

`n(1-p) = 150*(1-0.62)= 57>10`

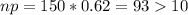
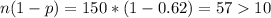
So then we can use the normal approximation and the distribution for the proportion is given:

`\hat p \sim N (p ,\sqrt{(p*(1-p))/(n)}`

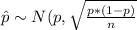
The estimated standard error is given by:

`SE= \sqrt{(0.62*(1-0.62))/(150)}=0.040`